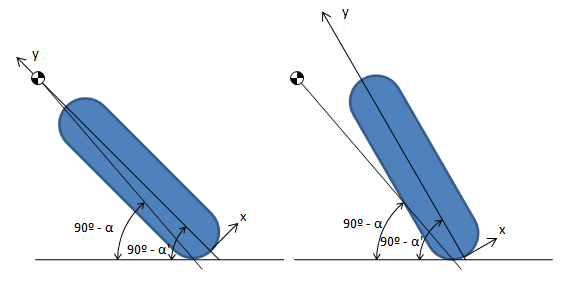
The lean angle is angle between line Center of Gravity (CoG) and contact patch the vertical vector of gravity
Theory of CoG displacement
In motorcycle racing, leaning the torso, moving the body, and projecting a knee or elbow to the inside of the turn relative to the bike.
This create a effect of displacement of CoG, in the next figure, we can see
For the same speed in the same turn α (lean angle) must the same to equilibrate forces. But with the Cog displacement the α' (banking angle) of motorbike, it doesn't necessary the angle
And the displacement of CoG is more close to tarmac (less in y axis) and external (more in x axis) of middle of motorcycle, α' (banking angle) is minor.
The typical explanation of this effect is mechanic, α' (banking angle) is limit in the motorcycle, the motorbike have a limit of the α' (banking angle), if you increase the difference α - α' value, you can pass more quick in the turn with the same α' (banking angle).
But this theory have one error, and this is the relationship of between weights.
If we make easy exercise with weight ratio, motorbike is 180kg, and pilot is 70kg, if the CoG is the same point in the circuit straight and pilot wants move overall CoG 1mm, the pilot must displace her CoG 3.57mm.
Therefore exchange the mechanic limit of α' baking angle motorbike to pilot, and the pilot touch tarmac and motorbike is able of turn more. I am sure that this image is reminded for a lot of people.
In this moment the limit es the pilot and the bike follow the turn, because touch with the other part lose the wheels.
I dont say that this action in the pilotage is wrong, I say that this explanation is not really true.
Theory of rolling cones
If we return the basic equation, we can see that the angle is equilibrium forces.Ans the RTurn is the radius of turn
If we suppose the wheel without deformation rear wheel 190/55R17 and front wheel 120/70R17.
But the wheel the radius cone is not radius wheel, this increase with the radius of wheel profile, and if we suppose this radio as rWheel
Hence if we compare the α' Bank angle and α Lean angle we reach this data
Cone semiangle is the same of α' banking angle, therefore if we displace the CoG, we can increase the cone radius and we can approach the rolling cone radius to equilibrium forces radius. and we need correct with the difference α - α' value
The problem is the high difference between angles, cone needs low angle, and for this reason the camber angle in the wheel car when it turns, the angle is low.

















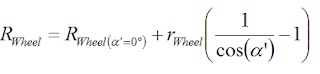





No hay comentarios:
Publicar un comentario